Mastering Data Analysis: How to Find the Range of a Data Set
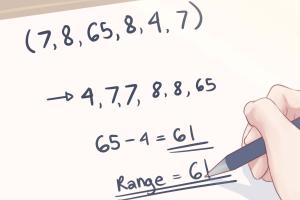
-
Quick Links:
- 1. Introduction
- 2. Understanding the Range
- 3. Importance of Finding the Range
- 4. How to Calculate the Range
- 5. Examples of Finding Range
- 6. Case Studies
- 7. Common Mistakes When Calculating Range
- 8. Advanced Topics in Range Analysis
- 9. FAQs
- 10. Conclusion
1. Introduction
The range of a data set is one of the most fundamental concepts in statistics. It provides a simple measure of the spread of the data and can offer critical insights into the variability of the data set. In this comprehensive guide, we will explore everything you need to know about finding the range of a data set, from the basic definition to advanced applications and common pitfalls.
2. Understanding the Range
The range is defined as the difference between the maximum and minimum values in a data set. Mathematically, the range can be expressed as:
Range = Maximum Value - Minimum Value
This simple formula can quickly provide insights into the dispersion of data, making it an essential tool for statisticians, data analysts, and researchers.
3. Importance of Finding the Range
Understanding the range of a data set is crucial for several reasons:
- Understanding Variability: The range helps illustrate how spread out the values are in a dataset, which can affect interpretations and decisions based on that data.
- Identifying Outliers: A large range may indicate the presence of outliers that could skew analysis.
- Informing Further Analysis: Knowing the range can guide analysts in deciding which statistical methods to apply when examining the data further.
4. How to Calculate the Range
Calculating the range is a straightforward process. Here's a step-by-step guide:
- Collect Your Data: Gather the data points you wish to analyze.
- Identify the Maximum Value: Determine the largest number in your data set.
- Identify the Minimum Value: Determine the smallest number in your data set.
- Apply the Range Formula: Subtract the minimum value from the maximum value to find the range.
For example, if your data set consists of the numbers 3, 7, 2, 8, and 5, the maximum value is 8, the minimum value is 2, and the range is:
Range = 8 - 2 = 6
5. Examples of Finding Range
Example 1: Simple Data Set
Consider the data points: 10, 15, 20, 25, 30. The maximum is 30, and the minimum is 10. Thus, the range is:
Range = 30 - 10 = 20
Example 2: Real-World Application
In a study measuring daily temperatures in a city over a week, the recorded temperatures were:
- Monday: 22°C
- Tuesday: 24°C
- Wednesday: 19°C
- Thursday: 26°C
- Friday: 23°C
The maximum temperature is 26°C, and the minimum is 19°C. Therefore, the range is:
Range = 26 - 19 = 7°C
6. Case Studies
To further illustrate the importance and application of range, let’s analyze a few case studies:
Case Study 1: Student Test Scores
In a classroom of 30 students, the scores on a mathematics test are as follows:
| Student | Score |
|---|---|
| 1 | 75 |
| 2 | 82 |
| 3 | 90 |
| 4 | 88 |
| 5 | 60 |
After calculating, the maximum score is 90, and the minimum is 60. Thus, the range is:
Range = 90 - 60 = 30
This significant range indicates a diverse level of understanding among the students.
Case Study 2: Monthly Rainfall
Consider a town's monthly rainfall over a year (in mm):
- January: 120
- February: 80
- March: 140
- April: 200
- May: 90
- June: 180
- July: 60
- August: 300
- September: 100
- October: 150
- November: 70
- December: 110
The maximum rainfall is 300 mm, and the minimum is 60 mm, giving a range of:
Range = 300 - 60 = 240 mm
7. Common Mistakes When Calculating Range
When calculating the range, several common mistakes can lead to inaccuracies:
- Overlooking Outliers: Failing to identify extreme values can skew the range significantly.
- Using Incorrect Data: Ensure the data set is complete and accurate before calculation.
- Misunderstanding the Definition: Remember that the range only considers the maximum and minimum values, not the distribution of the data.
8. Advanced Topics in Range Analysis
While the range is a basic statistic, it can be expanded into more complex analyses:
- Interquartile Range (IQR): This measure looks at the middle 50% of a data set and is less affected by outliers.
- Range in Multivariate Data: Understanding range in the context of multiple variables can provide deeper insights into relationships.
- Using Range in Data Visualization: Visual tools like box plots can effectively display the range among other data characteristics.
9. FAQs
Q1: What is the range of a data set?
The range is the difference between the maximum and minimum values in a data set.
Q2: How do you calculate the range?
Subtract the smallest value from the largest value in the data set.
Q3: Why is the range important?
The range provides a quick measure of data variability and can indicate the presence of outliers.
Q4: Can the range be negative?
No, the range is always a non-negative value since it is the difference between two values.
Q5: Is the range a good measure of dispersion?
While the range gives a quick overview, it can be influenced by outliers, so it is often used alongside other measures like the interquartile range.
Q6: How does the range compare to standard deviation?
The range measures total spread, while standard deviation quantifies how much individual values deviate from the mean.
Q7: What is the interquartile range?
The interquartile range (IQR) is the range of the middle 50% of the data, providing a better measure of central tendency without the influence of outliers.
Q8: Can the range be used for categorical data?
No, the range is applicable only for numerical data as it requires a meaningful way to quantify the difference between values.
Q9: How do you visualize the range?
Range can be visualized using box plots, which show the minimum, maximum, and quartiles of data.
Q10: What happens if the data set has identical values?
If all values are identical, the range will be zero, indicating no variability in the data set.
10. Conclusion
Finding the range of a data set is a vital skill in statistics and data analysis. Whether you are a student, a researcher, or a data analyst, understanding how to calculate and interpret the range can enhance your analytical capabilities. By following the methods outlined in this guide, you can make informed decisions based on the variability and distribution of your data.
Random Reads
- Connect laptop internet cell phone
- Easiest way to clear cache on roku device
- Download xbox games background
- How to make calls go directly to voicemail samsung galaxy
- How to reset water filter light whirlpool fridges
- How to replace ceiling fan pull chain switch
- How to replace bathroom tiles
- How to find drifloon pokemon diamond pearl
- How to find duplicates in excel
- How to replace a zipper pull