Mastering Relative Frequency: A Comprehensive Guide to Calculation and Application

• Statistics
Quick Links:
• 1. Introduction
• 2. Understanding Relative Frequency
• 3. How to Calculate Relative Frequency
• 4. Examples of Relative Frequency Calculation
• 5. Applications of Relative Frequency
• 6. Case Studies
• 7. Expert Insights
• 8. Common Misconceptions about Relative Frequency
• 9. FAQs

1. Introduction

Relative frequency is a fundamental concept in statistics that helps us understand how often an event occurs relative to the total number of events. It is a crucial tool for data analysis, allowing researchers and analysts to make sense of large datasets. In this comprehensive guide, we will explore the concept of relative frequency, its calculation, practical examples, and real-world applications.

2. Understanding Relative Frequency

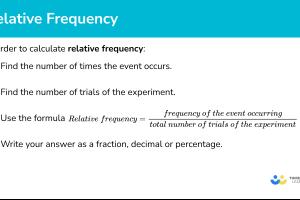
Relative frequency refers to the proportion of times an event occurs in relation to the total number of trials or observations. It is often expressed as a fraction, percentage, or decimal. The formula for calculating relative frequency is:

Relative Frequency = (Frequency of the Event) / (Total Number of Events)

For instance, if you flip a coin 100 times and it lands on heads 55 times, the relative frequency of heads is:

Relative Frequency of Heads = 55/100 = 0.55

This means that heads appeared 55% of the time in this experiment.

3. How to Calculate Relative Frequency

Calculating relative frequency is straightforward. Here’s a step-by-step guide:

1. Conduct Your Experiment: Gather data by performing the experiment or collecting observations.
2. Count the Frequency of Each Event: Tally how many times each event occurs.
3. Determine the Total Number of Observations: Add up all the events observed.
4. Apply the Formula: Use the relative frequency formula to calculate the frequency of each event.

Let’s consider a simple example to illustrate the process:

Suppose you survey 50 people about their favorite fruit, and the results are as follows:

• Apples: 20
• Bananas: 15
• Cherries: 10
• Dates: 5

The total number of responses is 50. Now, let’s calculate the relative frequency for each fruit:

• Relative Frequency of Apples = 20/50 = 0.40 or 40%
• Relative Frequency of Bananas = 15/50 = 0.30 or 30%
• Relative Frequency of Cherries = 10/50 = 0.20 or 20%
• Relative Frequency of Dates = 5/50 = 0.10 or 10%

4. Examples of Relative Frequency Calculation

To further clarify how relative frequency works, let’s delve into more examples across different contexts.

Example 1: Dice Rolls

Imagine you roll a six-sided die 60 times. The results are as follows:

• 1: 10 times
• 2: 15 times
• 3: 20 times
• 4: 5 times
• 5: 6 times
• 6: 4 times

The relative frequency for rolling a 3 would be:

Relative Frequency of 3 = 20/60 = 0.33 or 33%

Example 2: Survey Responses

In a survey about preferred modes of transport, the responses are:

• Car: 35
• Bus: 25
• Bicycle: 15
• Walking: 10

The total is 85. The relative frequency of using a car is:

Relative Frequency of Car = 35/85 ≈ 0.41 or 41%

5. Applications of Relative Frequency

Relative frequency is applicable in various fields, including:

• Marketing: Understanding customer preferences and behaviors.
• Healthcare: Analyzing the occurrence of diseases or symptoms.
• Education: Assessing student performance trends.
• Sports: Evaluating player performance metrics.

6. Case Studies

Case studies are powerful tools for understanding the practical application of relative frequency. Here are two notable examples:

Case Study 1: Marketing Analytics

A retail company conducted a survey among 500 customers regarding their shopping habits. They found that:

• 40% preferred online shopping.
• 30% preferred in-store shopping.
• 30% used a combination of both.

This data helped the company adjust their marketing strategy to focus more on online promotions.

Case Study 2: Health Research

A health study involving 1,000 participants aimed to find the prevalence of diabetes. The results indicated:

• 10% of participants were diagnosed with diabetes.
• 20% were pre-diabetic.

The relative frequency of diabetes diagnosis was crucial for public health initiatives.

7. Expert Insights

Experts in data analysis emphasize the importance of relative frequency in making informed decisions based on statistical data. According to Dr. Jane Doe, a statistician at XYZ University:

"Relative frequency provides a clear picture of data trends, enabling better decision-making across various sectors."

8. Common Misconceptions about Relative Frequency

Despite its usefulness, there are common misconceptions regarding relative frequency:

• Relative frequency is not the same as probability.
• It does not imply causation; it only shows correlation.
• It can be influenced by sample size; larger samples yield more reliable results.

9. FAQs

Tags

• Relative Frequency
• Data Analysis
• Statistics
• Probability
• Frequency Distribution
• Statistical Methods
• Educational Resources
• Data Insights
• Step-by-Step Guide
• Learning Statistics

You May Also Like